Image from System Matrix
This page is under construction - the Javascript calculation will be added.
The position of the image formed by a thick lens can be found by the matrix method. This involves multiplying a vector form of the incident vergence successively by matrices representing the refraction by the first surface, the translation to the second surface, and the refraction by the second surface. In this way the exit vergence is determined and from that vergence, the image distance.
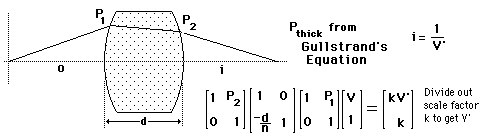
A thick lens can be characterized by its surface powers, its index of refraction, and its thickness.
|
The vergences can be calculated from the lens parameters and the object distance.The change in vergence when the light encounters a refracting surface is equal to the power of the surface:
Using the Cartesian sign convention, the object distance is typically a negative number since it points opposite to the direction of light travel.
| Object distance o = m |
Performing the indicated matrix multiplications gives the exit vergence from the thick lens.
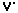 | = | 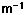 |
The exit vergence from the final surface determines the image distance with respect to that surface.
| Image distance i = m |
The equivalent power for the thick lens can be calculated from Gullstrand's equation:
 | = | 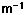 |
Note that the calculation does not take into account the change in lens thickness with the angle of the incoming ray. It is typical to do the calculation only for the paraxial rays where the departure from full thickness is negligible.
| Vergence Example | Matrix definitions | Example for two thin lenses |
Lens concepts
Thick lens concepts
| HyperPhysics***** Light and Vision | R Nave |