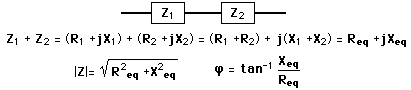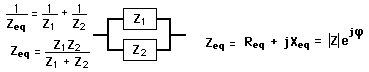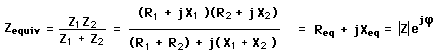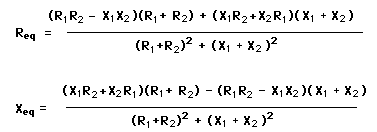Impedance

While Ohm's Law applies directly to resistors in DC or in AC circuits, the form of the current-voltage relationship in AC circuits in general is modified to the form:


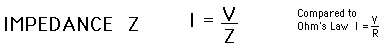
where I and V are the rms or "effective" values. The quantity Z is called impedance. For a pure resistor, Z = R. Because the phase affects the impedance and because the contributions of capacitors and inductors differ in phase from resistive components by 90 degrees, a process like vector addition (phasors) is used to develop expressions for impedance. More general is the complex impedance method.


Series and parallel combination of any two impedances
 Index

AC circuit concepts
  HyperPhysics***** Electricity and Magnetism Go Back





Impedance Combinations

Combining impedances has similarities to the combining of resistors, but the phase relationships make it practically necessary to use the complex impedance method for carrying out the operations. Combining series impedances is straightforward:

Calculation

Combining parallel impedances is more difficult and shows the power of the complex impedance approach. The expressions must be rationalized and are lengthy algebraic forms.

Expressions
 Index

AC circuit concepts
  HyperPhysics***** Electricity and Magnetism Go Back





Parallel Impedance Expressions

The complex impedance of the parallel circuit takes the form

when rationalized, and the components have the form

Calculation
 Index

AC circuit concepts
  HyperPhysics***** Electricity and Magnetism Go Back





Impedance Calculation

Impedances may be combined using the complex impedance method.

For
= + j
= + j

the series combination is

= + j= at phase.

The parallel combination is

= + j= at phase.

The units for all quantities are ohms. A negative phase angle implies that the impedance is capacitive, and a positive phase angle implies net inductive behavior.

Parallel impedance expressionsInductive reactanceCapacitive reactance
 Index

AC circuit concepts
  HyperPhysics***** Electricity and Magnetism Go Back