Variations in Difference Threshold
| Just noticeable difference | Decibels |
Sound level measurement
Reference
Backus
| HyperPhysics***** Sound | R Nave |
PhonsSaying that two sounds have equal intensity is not the same thing as saying that they have equal loudness. Since the human hearing sensitivity varies with frequency, it is useful to plot equal loudness curves which show that variation for the average human ear. If 1000 Hz is chosen as a standard frequency, then each equal loudness curve can be referenced to the decibel level at 1000 Hz. This is the basis for the measurement of loudness in phons. If a given sound is perceived to be as loud as a 60 dB sound at 1000 Hz, then it is said to have a loudness of 60 phons. The loudness of complex sounds can be measured by comparison to 1000Hz test tones, and this type of measurement is useful for research, but for practical sound level measurement, the use of filter contours has been commonly adopted to approximate the variations of the human ear.
|
Index Sound level measurement | ||
|
Go Back |
SonesThe use of the phon as a unit of loudness is an improvement over just quoting the level in decibels, but it is still not a measurement which is directly proportional to loudness. Using the rule of thumb for loudness, the sone scale was created to provide such a linear scale of loudness. It is usually presumed that the standard range for orchestral music is about 40 to 100 phons. If the lower end of that range is arbitrarily assigned a loudness of one sone, then 50 phons would have a loudness of 2 sones, 60 phons would be 4 sones, etc. |
Index Sound level measurement | ||||||||||||||||||||||||
|
Go Back |
Decibel CalculationThe sound intensity in decibels above the standard threshold of hearing is calculated as a logarithm. If the intensity as a multiple of threshold is |
Index Sound level measurement | ||
|
Go Back |
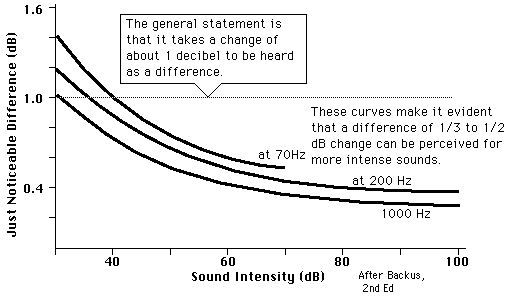
JND in Sound IntensityA useful general reference is that the just noticeable difference in sound intensity for the human ear is about 1 decibel. In fact, the use of the factor of 10 in the definition of the decibel is to create a unit which is about the least detectable change in sound intensity. That having been established, it can be noted that there are some variations. The jnd is about 1 dB for soft sounds around 30-40 dB at low and midrange freqencies. It may drop to 1/3 to 1/2 a decibel for loud sounds.
|
Index Sound level measurement | ||
|
Go Back |
Variations in Difference Threshold
|
Index Sound level measurement Reference Backus | ||
|
Go Back |