Force and Momentum

The net external force acting on an object can be evaluated as the rate of change of momentum. This turns out to be a more fundamental way of stating the force than the use of Newton's second law. Using Newton's second law and momentum:

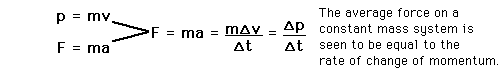
But this limited relationship can be generalized to
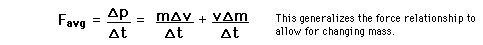
and further generalized by calculus methods to include instantaneous rates of change
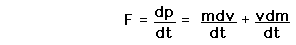This formulation of the force relationship permits varying mass, as in rocket propulsion.

In the language of calculus, we say that the force is defined as the derivative of the momentum. The process above involves the product rule for derivatives.

Index

Limits on Newton's 2nd law
 
HyperPhysics***** Mechanics ***** Newton's laws R Nave
 Go Back





Rocket Propulsion

The thrust of a rocket can be modeled from a generalization of Newton's 2nd Law to include a variable mass:

In free space this would lead to a rocket velocity
Show

and for a rocket launched from the Earth's surface it leads to the expression
Show

at low altitudes where g can be considered to be constant.
Index
 
HyperPhysics***** Mechanics R Nave
 Go Back





Thrust of a Rocket

Rocket thrust results from the high speed ejection of material and does not require any medium to "push against". Conservation of momentum dictates that if material is ejected backward, the forward momentum of the remaining rocket must increase since an isolated system cannot change its net momentum.
Show
Index

Rocket propulsion
 
HyperPhysics***** Mechanics R Nave
 Go Back





Thrust of a Rocket


Rocket thrustShow differential approachFurther discussion of rocket thrust
 Index

Rocket propulsion
 
HyperPhysics***** Mechanics R Nave
 Go Back





Thrust of a Rocket


Rocket thrustShow algebra approachFurther discussion of rocket thrust
 Index

Rocket propulsion
 
HyperPhysics***** Mechanics R Nave
 Go Back





Rocket Thrust Discussion

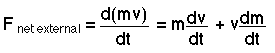
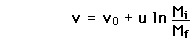
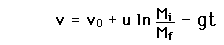
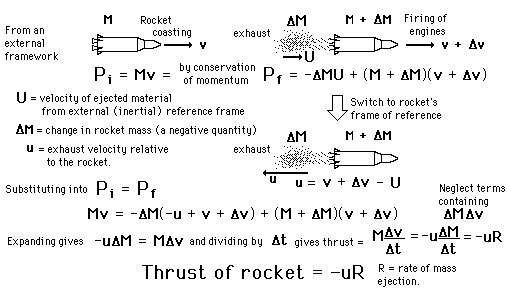
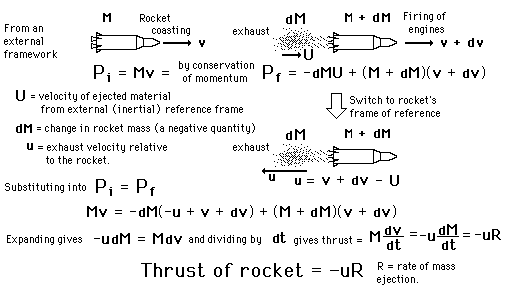
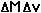Developing the expression for rocket thrust involves the application of conservation of momentum in an accelerating reference frame. It can be developed in an approximate fashion using finite differences and algebra. This requires neglecting terms like . When the appropriate limits are taken to get an instantaneous expression, these approximations disappear since the quantities dM and dt approach zero.
Show algebra approachShow calculus approach
 Index

Rocket propulsion
 
HyperPhysics***** Mechanics R Nave
 Go Back