Simplified Model: Sound Reinforcement

Assume*: Inverse square law dropoff of sound Omnidirectional loudspeakers and microphones

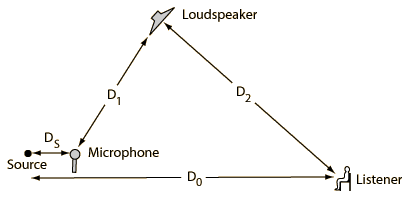
The loudspeaker provides more sound to the listener than would otherwise have been received, but it also produces sound at the location of the microphone. This feedback to the microphone limits the amount of amplification which can be used.

The microphone creates an electrical image of the sound which is amplified and used to drive a loudspeaker.

*These idealizations are never met in any real room, but they provide a framework for building a model. The assumptions will be relaxed later.

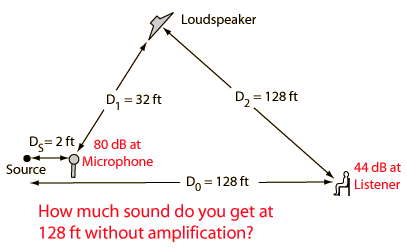
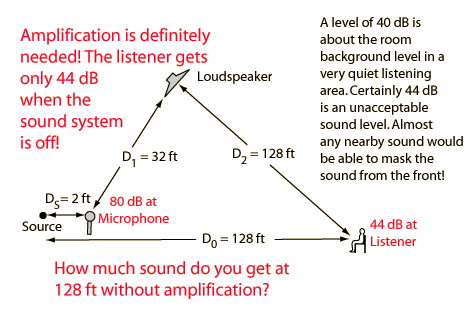
Numerical Example: Need for Amplification

Numerical Example: Need for Amplification

Maximum Amplification Condition

When the feedback to the microphone is equal to the original sound input level, the gain can no longer be increased without an uncontrolled increase in output, usually in the form of a loud ring at one frequency. Practically, the sound should be kept about 3 dB below that point. If 80 dB is the input level to the microphone, then 80 dB feedback return is an upper bound. Using the inverse square law, the resulting levels are as shown.