Radiated Power from Blackbody

When the temperature of a blackbody radiator increases, the overall radiated energy increases and the peak of the radiation curve moves to shorter wavelengths. When the maximum is evaluated from the Planck radiation formula, the product of the peak wavelength and the temperature is found to be a constant.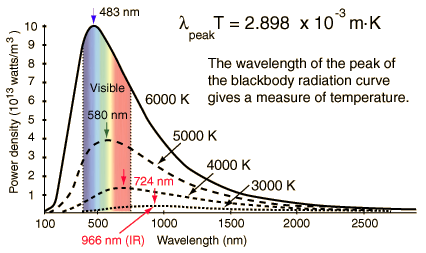
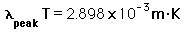Calculation

The total power radiated by a blackbody is given by the Stefan-Boltzmann equation, but it is often interesting to know the fraction of power which is emitted in the visible or some other wavelength range.

Temperature T = K = °C

Area A = cm2 = x10^ m2

Emissivity = (e = 1 for ideal radiator)

The total power radiated is P = watts = x10^ watts.

Finding the power radiated within a given wavelength range requires integration of the Planck radiation formula over that range. The radiated power per unit area is the Planck energy density multiplied by c/4. It can be approximated numerically by taking a sum of values of the Planck radiation density times a wavelength interval. The result of dividing the wavelength interval into 100 steps is as follows.

For the wavelength range l1 = nm to l2 = nm,

The radiated power is Pinterval = watts = x10^ watts.

This is % of the total radiated power.

The radiated power in a given wavelength interval Dl at wavelength l can be approximated by

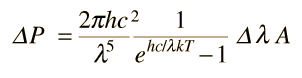
The above approximate calculation for the radiated power in a chosen wavelength range is a brute force sum over 100 terms formed by dividing the specified wavelength range into 100 parts. This is then compared to the total radiated power calculated from the Stefan-Boltzmann equation. You can check out the calculation by choosing the wavelength range so that it covers essentially all the radiated energy. You will find that the calculation diverges if you pick a starting wavelength too close to zero, and if you put in too large a wavelength. But by examining the wavelength range for which the radiated power appears to be significant, you can choose reasonable limits on wavelength and confirm that you get essentially all the radiated power.

Index

Blackbody radiation concepts
 
HyperPhysics***** Quantum Physics R Nave
 Go Back