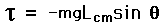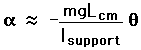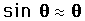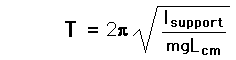Physical Pendulum
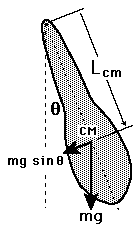 |
Hanging objects may be made to oscillate in a manner similar to a simple pendulum. The motion can be described by "Newton's 2nd law for rotation":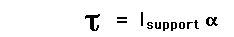
|
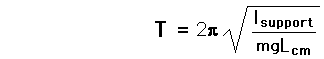
| Calculation | Compare to simple pendulum |
| Rod pendulum | Circular geometries | Combinations |
Periodic motion concepts
| HyperPhysics***** Mechanics | R Nave |