Graham's Law
When gases are dissolved in liquids, the relative rate of diffusion of a given gas is proportional to its solubility in the liquid and inversely proportional to the square root of its molecular mass. Important in the transport of respiration gases is the relative diffusion rate of oxygen and carbon dioxide in the plasma of the human body. Carbon dioxide has 22 times the solubility, but is more massive (44 amu compared to 32 for oxygen). According to Graham's law, the relative rate of diffusion is given by
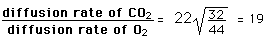
| Diffusion | Osmosis | Membrane transport | Henry's Law | Fick's Law |
Kinetic theory concepts
Applications of kinetic theory