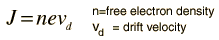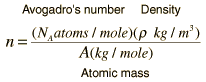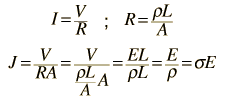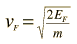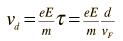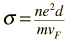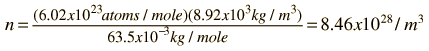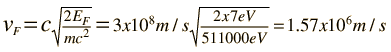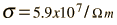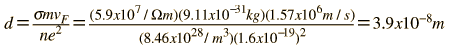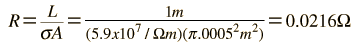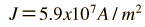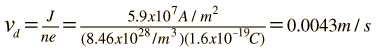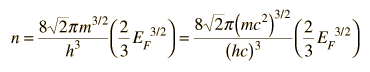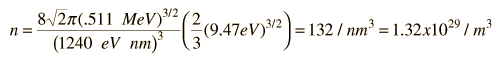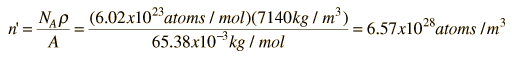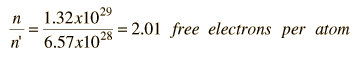Microscopic View of Copper Wire
As an example of the microscopic view of Ohm's law, the parameters for copper will be examined. With one free electron per atom in its metallic state, the electron density of copper can be calculated from its bulk density and its atomic mass.
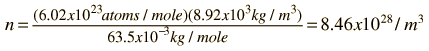
The Fermi energy for copper is about 7 eV, so the Fermi speed is
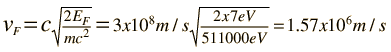
The measured conductivity of copper at 20°C is
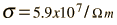
The mean free path of an electron in copper under these conditions can be calculated from
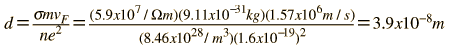
The drift speed depends upon the electric field applied. For example, a copper wire of diameter 1mm and length 1 meter which has one volt applied to it yields the following results.
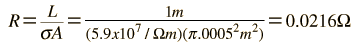
For 1 volt applied this gives a current of 46.3 Amperes and a current density
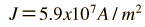
This corresponds to a drift speed of only millimeters per second, in contrast to the high Fermi speed of the electrons.
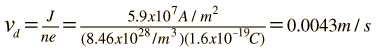
Caution! Do not try this at home! Dr. Beihai Ma of Argonne National Laboratory wrote to point out that the current density of 5900 A/cm2 in this example is over ten times the current density of 500 A/cm2 that copper can normally withstand at 40°F. So doing this in the laboratory might be too exciting. Thanks for the sanity check Dr. Ma.
(If you scale down the voltage applied so that the current is just 3 Amperes, current density 382 A/cm2, so that the copper wire will remain intact, the calculated drift velocity is just 0.00028 m/s. This would be more typical for working conditions in this wire. )
|