The Wave Equation

The wave equation for a plane wave traveling in the x direction is

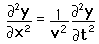
where v is the phase velocity of the wave and y represents the variable which is changing as the wave passes. This is the form of the wave equation which applies to a stretched string or a plane electromagnetic wave. In two dimensions, the wave equation takes the form

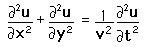
which could describe a wave on a stretched membrane.

Waves in Ideal String

The wave equation for a wave in an ideal string can be obtained by applying Newton's 2nd Law to an infinitesmal segment of a string.

If a constant horizontal tension T is maintained in the string, then Newton's 2nd law becomes Combination of these two expressions for small angles gives Show details Solutions to wave equation

String Wave Equation Development

Analysis of the forces on a segment of stretched string gives two relationships:

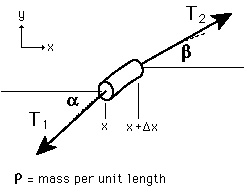
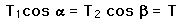
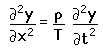
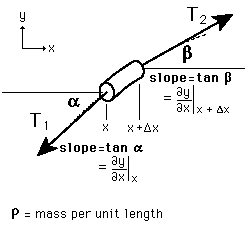
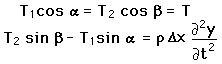
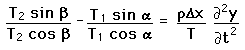
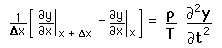
Combining gives and relating this to the slopes at the segment ends gives In the limit this becomes Wave equation

Ideal String Constraints

In order for the wave equation to apply to the waves in a string, it must meet certain constraints. For an ideal string, it is assumed that

1. The string is perfectly uniform with a constant mass per unit length, and is perfectly elastic with no resistance to bending.

2. The string tension is presumed to be large enough so that gravity can be neglected.

3. Small segments of the string are presumed to move transversely in a plane perpendicular to the string, and that the displacements and slopes of segments of the string are small.

Though stringent, these idealizations permit the development of a wave equation which describes well the vibrations of thin real strings.